Summary: In many fields, the ‘greatest’ (be they philosophers, playwrights, composers, etc.) are selected disproportionately more from those who lived in the distant past. I speculate as to what might be driving this bias towards ‘ancient greatness’, but one important takeaway is that we can be confident that the greatest of the past are likely inferior to the greatest amongst us today in terms of ‘innate ability’. So perhaps we should not regard them so highly.

[Very rough draft: advice/criticism on data, analysis, or style welcome, as is advice on whether this is worthy of going into academia, and if so how. Thanks to Rob Wiblin, Will Crouch, Catriona McKay, and Sam Bankman-Fried for ideas/prior discussion.]

Introduction

If you look at a field of human endeavour (mathematics, philosophy, the arts, military strategy – pretty much anything) the reputedly ‘greatest ever’ in these fields have tended to live in the distant past.
Take philosophy as an example. Polls of the ‘greatest philosopher’ of all time broadly agree on a corpus of ‘ancient greats’. Here’s the top ten from a poll from Brian Leiter’s philosophy blog, with their dates added:

1. Plato (428-348 BC)
2. Aristotle (384-322 BC)
3. Kant (1724-1804)
4. Hume (1711-1776)
5. Descartes (1596-1650)
6. Socrates (469-399 BC)
7. Wittgenstein (1889-1951)
8. Locke (1632-1704)
9. Frege (1848-1925)
10. Aquinas (1225-1274)

The list is dominated by ancient Greeks and enlightenment Europeans, with only a couple of thinkers in the last couple of centuries getting a look in. I think Leiter’s poll (modulo some quibbles with the exact ordering) broadly captures the consensus view amongst who are the greatest philosophers, at least in the western tradition.

On the face of it, having the ‘greatest ever’ philosophers spread out from antiquity to the present day seems plausible. But consider how the human population has grown over time (data from Wikipedia):

The population has grown dramatically, so if we think about birth order rather than time, the greatest philosophers were generally among those born first. Why?

Great philosophers are neither distributed in proportion to population, nor uniformly by birth order
Pretend for argument’s sake that philosophical greatness was solely a matter of innate ability, and the amount of innate ability varies randomly from person to person. If that was so, you would expect the most able (and therefore the greatest) philosophers to be distributed randomly by birth order – everyone, whether born first, billionth, or last, has the same (remote) chance of being in the top X of people by philosophical ability. When looking at groups of people, we should take the incidence of ‘great philosophers’ (at whatever level of greatness we are interested), to be proportional to the size of that group: bigger groups have more tickets in this natural lottery, and so are more likely to have lucky winners amongst them. So we should expect the greatest philosophers to be distributed across history in a similar manner to population size over history, and as more recent times have more people in them, we should expect more of the greatest philosophers to be in these times than the distant past.

We can investigate this graphically. First, give each philosopher in the ‘top twenty of all time’ a score for how high they come in the ranks (Plato gets 20, second place Aristotle gets 19, and so on)[ref]You may worry about whether we can really set these ordinal ranks on this cardinal scale. I have played with discarding the ranking data (giving each philosopher, no matter their rank, the same weight), and this hasn’t really changed the results)[/ref]. Now plot them by score and date of their birth:

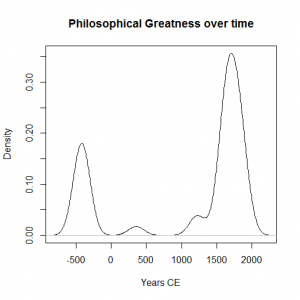
By its nature, this data is noisy and low count. What we’d like to do is smooth these points into a nice contour over time to give a ‘density’ of greatest philosophers across history. Using a Gaussian kernel results in the following:

Now compare this to the population graph given before, plotted across the years 500 BCE to 1900 CE:[ref]A problem, which I’ll come back to later, is how to ‘window’ our look at greatness: people living in prehistory or the present day have very little chance of being recognized as an ‘all time greatest X’. I have in subsequent analysis windowed by the earliest and latest greatest person on the list. For more discussion, see later.[/ref]

It doesn’t look like these lines marry up well at all. There’s a surge of great philosophers at 400-300 BC despite the very low population, and although both great philosophers and population have a higher incidence towards the end of the graph, the rise is well out of sync. Great philosopher density peaks and declines whilst population is still surging – here’s the figure ‘zoomed in’ on years 1400-1900 to make that clearer:

Performing some inferential statistics on how poorly these curves match is beyond me (suggestions welcome!), but there’s another approach: if we expect great philosophers to be uniformly distributed by birth order, we can test the incidence of great philosophers by birth order against the uniform distribution.

The snag is that getting reliable data on cumulative population (i.e. the number of people that have ever been born by a given date), and this is extremely difficult. Some brave folks at the population research center have tried to do just this. We can use this data (with some linear interpolation) to give approximate birth ranks for the great philosophers out of those born between years 500 BCE – 1900 CE, and then see if this looks like a uniform distribution as anticipated by a natural lottery.[ref]One correction that should be made is trying to look instead at “all those born who lived beyond 15 years of age, given the very high child mortality in the past. This could in principle be estimated by adjusting the PRC model, which I might do if people find this interesting.[/ref]

Here’s a density plot of great philosophers by birth rank over 500 BCE to 1900 CE, with the ranks normalized to the [0,1]  interval:

This is highly non-uniform (P < 0.001 ; one sample K-S test against a uniform distribution), so the natural lottery hypothesis isn’t doing too well for philosophical greatness.

Excursus: Do any fields fit with ‘natural lottery’ accounts of greatness?

It would be interesting to see if the greatest in any fields follow what the natural lottery would expect. The main limiting factor is getting reliable measures of greatness: ratings by a single person are often idiosyncratic, and ‘objective’ metrics are very hard to find. Here are some I found a useful ranking to play with:

Writers

There’s a world library list of the ‘hundred greatest books’ of all time, conducted by polling 50 or so living authors. With books one should expect some degree of clustering (the greatest writers can write multiple great books), making the ‘birth rank’ analysis illegitimate, one would still expect, if great writers were distributed by a natural lottery, the greatest books should (modulo significant noise) have a density proportional to population over time.

Doing similar analysis to previously, we get a graph like this (population is the red line):

This looks a good fit for the natural lottery hypothesis, although there is a similar (albeit milder) worry if we zoom in on more recent history, with a declining trend of great books whilst population is in the midst of exponential growth. However, ‘peak great books’ lies pretty close to the modern day (1900 or so), making this discrepancy easier to explain away.

Physicists

Physics world surveyed 100 leading physicists to give a ‘top ten of all time‘ list:

1. Einstein
2. Newton
3. Maxwell
4. Bohr
5. Heisenberg
6. Gallileo
7. Feynman
8. Dirac
9. Schrodinger
10. Rutherford

Doing the same as before, we get:

Given the small sample size, this is a fair fit to a natural lottery.

‘Greatest minds’

A community driven polling website (ranker.com) has a list of ‘greatest minds of all time’. Although the voters and rankers are non-expert, the size of people involved might make it a fair reflection of the ‘commensense view’ of the greatest thinkers ever. Doing the same as previously with the top 100 gives this:

This looks very similar to the philosophers data given previously, right down to the ‘early modern greats’ giving a peak and decline whilst population is taking off:

The greatness of the old becomes even more surprising when accounting for other factors

So the natural lottery hypothesis doesn’t really fit the data, which shows ‘greatness’ seems to be distributed further in the past than the model will predict.

We’d expect a lot of factors to intervene between innate ability and being regarded as a great philosopher (or great mind, physicist, et c.)  Some of these factors would amount to (time-invariant) noise, and including these does not change the hypothesis or how surprising this evidence is: “natural lottery” or “natural lottery with extra sources of variation” predict basically the same thing.[ref]One factor is there seems a lot of clustering (Early modern Europe, Ancient Greece, et c., but over time this clustering should fall out of the model, the chances of living in a golden age or dark age should either not correlate with time, or there should be increasing chance of living in a golden age in more recent times, because of the reasons listed below.[/ref] We should concern ourselves with systematic factors that tend to go ‘one way’. As far as I can see, trying to include these makes the over-representation of “old greats” more surprising.

Nature

The natural lottery model assumes everyone, no matter when they are born, has an equal albeit remote chance of having ‘greatest ever’ innate ability at a given field. Yet we should think that, given improvements in nutrition, living conditions, and medicine, that children born in more recent years should be (amongst other things) smarter than those in ancient times, which should make them more likely to have those with ‘greatest ever’ innate abilities. (Cf. the Flynn effect)

Nurture

Even if one has stupendous innate ability, a lot of factors intervene from there to becoming a ‘greatest ever’. It looks like the modern world should be much better at nurturing the innately able into elite performance, and the ancient would should be much more prone to ‘losing’ its geniuses.

1. The risk of dying as a child (or dying ‘before one’s time’) was much higher in the past.
2. A number of fields seem to have pre-requisites (e.g. literacy for being a great philosopher), as factors like worldwide literacy, numeracy, and fraction educated to primary school level have all improved over time, more people have these pre-requisites in place.
3. There were greater barriers to achievement in the past, due to factors like slavery, strict class systems, lack of individual rights and liberty, and so forth. The highly able born into disadvantaged groups in modern times have a much greater chance of ‘making it’ than those born into similar groups in antiquity.
4. It seems pretty plausible that becoming a great in a particular field also requires a lot of hard work to develop ones innate talents. Those in the modern world have advantages here in that they are more likely to be able to devote more of their time to their field (instead of subsistence farming, military service, et c.), and there are now better tools available for them to use for their own development (computers, wider access to information, et c.)
5. Increasing knowledge over time allows people living in more modern times to be better informed (and, if you buy moral progress, more morally enlightened) which may have knock on benefits to doing good science or philosophy.

Biases in the perception of greatness

From all of this, the ‘greatest in history’ should be distributed closer to the present than a natural lottery would predict, rather than further into the past. Explaining phenomena as complicated as this is extremely unreliable, but I would put the plurality of my credence in the following ideas to explain why the old greats are ‘punching above their weight’ compared to their actual ability.

Low-hanging fruit

Fields of knowledge are often hierarchical, with more difficult developments being built on top of easier ones. I’d guess euclidean geometry is much less difficult than modern academic work in the field, and the fact that the discoveries of the ‘old greats’ are often taught earlier in school suggests they are easier to grasp than what comes afterwards (I can just about do some basic problems in Newtonian mechanics thanks to my schooling – I cannot do any ‘basic’ problems in General Relativity).

If so, you might hypothesize that these ‘earlier’ discoveries are easier to make, and so those with (relatively) lesser ability can make them provided they are fortunate enough to be born at a time where these lower-hanging fruit are not plucked. This would act to inflate the reputed greatness of the old greats.

Polymath premium and the ‘Forefather’ effect

People who make significant advances in a number of fields appear much more impressive than those who achieve in only one, likewise those whose work gives rise to an entirely new field of endeavour. Whitehead’s remark that western philosophy is ‘Footnotes to Plato’ suggests that Plato is a superlative genius if he has covered so much ground in philosophy by himself. I guess there are similar sentiments when thinking of people like Da Vinci or Goethe.

Yet (given low-hanging fruit), both of these things are easier to do earlier in human history, where there were much lower heights to scale before one was at the margin of human knowledge, and much less ground had been explored. It is difficult to imagine a modern day scientist making important breakthroughs across several fields, simply because scientists have had to divide labour more and more by specialism (and sub-specialism) to give the requisite mastery required to make new discoveries. Compare this to the sum total of relevant knowledge in ancient Greek times.

Thus the old greats had the opportunity to christen new fields by themselves or range across disciplines, but in doing so made it incrementally harder for subsequent, equally able people, to do the same.

Retroactive esteem

The old greats may also accrue greatness retroactively through the deference (and reference) paid to them by their successors. Almost all of my knowledge of Aristotle comes from secondary sources, but this makes Aristotle this ‘larger than life’ figure with a presence across philosophy. (The fact that his name also names a fairly common philosophical view also helps, and this effect applies to similar ancient greats who get views of phenomena named after them).

Difficulty judging greatness within ‘living memory’

Another problem is that people are reluctant to call recent people all time greats, perhaps because our assessments of greatness ‘in our own time’ are less reliable than looking back: we might be confident Terrence Tao or Stephen Hawking are world leading, yet be reluctant to rank them amongst the ancients yet. I have tried to compensate for this by setting the comparison ranges between population and greatness to the earliest and latest great person (so avoiding the most recent years where no one is yet considered great), but you would still expect some tail off towards the most recent part of the interval, which is what we observe in all the different categories (although the interval varies, with philosophy having a longer ‘tail off’ period than books, for example).

Smaller ponds in antiquity

It is fairly plausible that we view greatness as positional ‘within eras’, but are much less good at judging between them. So we look for the ‘greatest few thinkers of antiquity’, and assume they’re on a par with the ‘greatest few thinkers of the modern day’. Yet antiquity is a much smaller pond than the modern day, and so you don’t need to be so able to be the greatest of this group.

Why Athens has 13 Platos, and Europe 4 Kants

Let’s assume Plato was the most able philosopher in ancient Greece when he lived (due to winner’s curse, not that likely). Let’s also pretend philosophical ability is solely a function of intelligence. How smart would we expect him to be?

The population of Attica in Plato’s time was somewhere around 300 000 (only 60 000 were thought to be men who could vote, the rest being women or slaves, but ignore this). If Plato is the smartest, that puts him at ‘one in 300 000 level’, around 4.2 sigma.

Modern Athens has a population of 4 million people. You’d expect around 13 people in modern Athens to have ‘Plato level’ 1-in-300 000 intelligence. It’s possible Plato would be top dog in this group, but it is a priori unlikely (1/14). You’d expect the most able philosopher in Athens to be about a standard deviation smarter than Plato (granting normality).

But the modern world has much more than Athens. The population of Europe and the United states is just over 1 billion people (1035 million). So we’d expect just over three and a half thousand people with ‘Plato-level’ philosophical ability. The chances of Plato being the most able among this group is minuscule. The smartest person would be expected to be two standard deviations better than Plato: if philosophical ability was solely a matter of intelligence, that’s about the difference between someone in MENSA and someone with average intelligence.

The same applies (to a lesser extent) looking at the not-so-ancient greats: there were 164 million people in Europe in the time of Kant, and 800 or so now, so we’d expect around 4 ‘Kant level’ philosophers, granting that Kant was the greatest philosopher in Europe in his time.

We shouldn’t take this analysis too seriously (besides all the liberties taken, a lot depends on how you pick your comparison classes: was Plato the best in Athens, or the best in the ancient world?)[ref]A related concern is this sort of analysis begins to look like the reverse gambler’s fallacy. Toy reductio: Wittgenstein was the smartest guy in his primary school of 20, I’m the smartest guy in my school of 400, so I’m very likely smarter than Wittgenstein: correct if that is all we have to go on, but there are plainly more sources of information available to us. However, when the comparison classes get big enough, these sorts of worries fade. And although the greatest philosopher of all time is as likely to fall in the ancient athenians by natural lottery than any other group of 300 000, getting #1, #2 and #7 in this group (Plato, Aristotle, Socrates) is implausible.[/ref] But the general point is this: even if we ignore all the advantages modernity brings to cultivating exceptional ability, we should expect by the natural lottery alone many more people living now having abilities matching or exceeding the ancient greats – or even the fairly modern greats. It is possible that if Plato was born today rather than in ancient Athens, instead of being the ‘greatest philosopher of all time’, he’d be struggling to get tenure.

On being less deferential to the past

Does any of this matter? The fact that ‘historical luck’ played a large part of what made the ancient greats so great does not make their achievements less significant. That a lot of what made Dostoevsky one of the greatest in world literature had plausibly more to do with living in the right place, at the right time, and among the right people, rather than his own (still incredible) ability does not make The Brothers Karamazov less wonderful, even if we anticipate there should be even more talented writers among us today. Similarly, the significant milestones in philosophy or science are still significant and venerable, even if the names we associate with them are there more by historical accident ‘best in human history’ talent.

It does mean, though, we should pay less deference to the achievements (and achievers of the past). Instead of the vast secondary literature to try and find a charitable account of Socrates techne based refutation of Thrasymachus at the start of Plato’s Republic (because Plato and Socrates, intellectual titans they are, would not give a bum argument) we should trust our judgement that this was just a bad argument, because Plato and Socrates were not that brilliant, and were living in comparatively unenlightened times, and so our prior on them just making a mistake should not be that low. More generally, much of our scholarly emphasis on the old greats is probably misplaced if this enterprise is motivated (in part) by the hopes of excavating some hidden gems of insight in their work – they’re much less likely to have insight relevant to our current state of knowledge than modern day thinkers.

It also means we should be less down on modern work compared to the ancient greats. It isn’t completely crazy (pace adverse selection) to think there are some people writing fanfiction as talented as Shakespeare, or that TV serials may turn out to have similar merit to War and Peace, or that Reasons and Persons is more than a match for The Critique of Pure Reason. And even if the ancients have taken more than their fair share of historical greatness, making it much harder for those who came later, we should be really confident that our current crop of scientists, philosophers, and creatives are superior to those of any age that has come before. It’s a great time to be alive.

Raw data:

Here (inc. graphs, raw data, and R workspace and history (not cleaned up for my frequent inability to write R code with correct syntax)